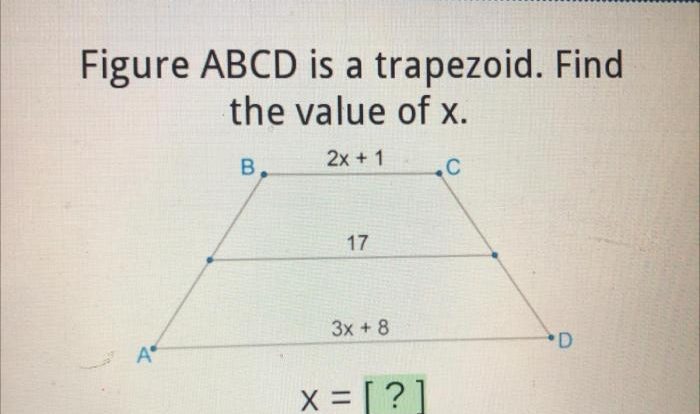Beginning with the building block of math crossword clue, this discourse delves into the fundamental components that constitute the very essence of mathematics. These building blocks, the cornerstone of mathematical operations, provide the framework for understanding the intricate world of numbers, equations, and structures.

From the rudimentary elements of numbers and variables to the more complex operators and relationships, this exploration unravels the interplay between these building blocks, revealing their significance in shaping mathematical outcomes.

Building Blocks of Mathematics: Building Block Of Math Crossword Clue

The building blocks of mathematics are the fundamental concepts and operations that form the foundation of the subject. These include numbers, sets, operations, and relations. They are the basic elements that are used to construct more complex mathematical structures and solve problems.

paragraphNumbers are the most basic building block of mathematics. They are used to represent quantities and to perform operations such as addition, subtraction, multiplication, and division. Sets are collections of objects that share a common property. They are used to organize and classify objects and to perform operations such as union, intersection, and complement.

Operations are actions that are performed on numbers or sets. They include addition, subtraction, multiplication, division, and exponentiation. Relations are relationships between sets or between elements of a set. They include equality, inequality, and order.

Numbers

• Natural numbers: 1, 2, 3, …
• Integers: … -3, -2, -1, 0, 1, 2, 3, …
• Rational numbers: Numbers that can be expressed as a fraction of two integers, a/b, where b is not zero.
• Irrational numbers: Numbers that cannot be expressed as a fraction of two integers.
• Real numbers: The set of all rational and irrational numbers.

Sets, Building block of math crossword clue

• A set is a well-defined collection of distinct objects.
• Sets are denoted by braces .
• The elements of a set are separated by commas.
• The order of the elements in a set does not matter.
• The same element cannot appear more than once in a set.

Operations

• Addition: The operation of combining two or more numbers or sets to get their sum.
• Subtraction: The operation of finding the difference between two numbers or sets.
• Multiplication: The operation of combining two or more numbers or sets to get their product.
• Division: The operation of finding the quotient of two numbers or sets.
• Exponentiation: The operation of raising a number to a power.

Relations

• Equality: The relation between two objects that have the same value.
• Inequality: The relation between two objects that do not have the same value.
• Order: The relation between two objects that are arranged in a specific order.

Types of Building Blocks

The fundamental building blocks of mathematics form the cornerstone of mathematical expressions and equations. These building blocks include numbers, variables, and operators, each playing a distinct role in mathematical operations.

Numbers

• Numbers represent specific quantities and serve as the basic units of mathematical operations.
• Examples of numbers include integers, rational numbers, irrational numbers, and complex numbers.

Variables

• Variables represent unknown or changing quantities in mathematical expressions and equations.
• They are typically represented by letters, such as x, y, or z.
• Variables allow for the generalization of mathematical statements and the exploration of relationships between quantities.

Operators

• Operators are symbols that indicate the operations to be performed on numbers or variables.
• Examples of operators include addition (+), subtraction (-), multiplication (*), division (/), and exponentiation (^).
• Operators determine the order of operations and the result of mathematical expressions.

Operations and Relationships

In mathematics, building blocks are not merely isolated entities but are combined and manipulated through mathematical operations. These operations serve as the tools that allow us to transform, analyze, and derive new insights from the building blocks.

The relationships between different building blocks are crucial in determining the outcomes of mathematical operations. These relationships can be additive, subtractive, multiplicative, or divisive, and they dictate how the building blocks interact with each other.

Arithmetic Operations

• Addition: Combines two or more numbers to produce their sum.
• Subtraction: Finds the difference between two numbers.
• Multiplication: Multiplies two or more numbers together.
• Division: Divides one number by another.

Algebraic Operations

• Exponents: Raises a number to a specified power.
• Logarithms: Finds the exponent to which a base must be raised to produce a given number.
• Radicals: Extracts the nth root of a number.

Impact of Relationships

The relationships between building blocks can have a profound impact on mathematical outcomes. For example, the order of operations in arithmetic can change the result of a calculation. Similarly, in algebra, the relationship between variables and constants determines the behavior of equations and functions.

Mathematical Structures

Building blocks of mathematics can be organized into mathematical structures, which are abstract frameworks that provide a way to understand and describe mathematical concepts. These structures include sets, groups, rings, and fields, each with its own properties and applications.

Sets, Building block of math crossword clue

A set is a well-defined collection of distinct objects. Sets are used to represent collections of elements that share common characteristics. The elements of a set can be any type of mathematical object, such as numbers, variables, or even other sets.

• Properties of sets include union, intersection, complement, and subset relations.
• Sets are used in various mathematical domains, including set theory, logic, and computer science.

Groups

A group is a non-empty set together with an operation that combines any two elements of the set to form a third element of the set. Groups are used to study symmetry and transformations.

• Properties of groups include associativity, identity element, and inverse elements.
• Groups are used in various mathematical domains, including algebra, geometry, and number theory.

Rings

A ring is a non-empty set together with two operations that combine any two elements of the set to form a third element of the set. Rings are used to study algebraic structures and number systems.

• Properties of rings include associativity, distributivity, and identity elements.
• Rings are used in various mathematical domains, including algebra, number theory, and algebraic geometry.

Real-World Applications

The building blocks of mathematics find widespread applications in various real-world contexts. Their significance extends beyond the realm of academia, playing a crucial role in fields such as science, engineering, and finance.

In science, mathematical principles provide the foundation for understanding and predicting natural phenomena. For instance, in physics, the laws of motion and gravity are expressed using mathematical equations. These equations allow scientists to calculate the trajectory of objects, predict the behavior of fluids, and design structures that can withstand external forces.

Engineering

In engineering, mathematics is indispensable for designing and constructing everything from bridges to aircraft. Engineers use mathematical models to simulate and analyze complex systems, ensuring their safety, efficiency, and durability. For example, in civil engineering, mathematical calculations are used to determine the load-bearing capacity of structures, ensuring they can withstand earthquakes and other natural disasters.

Finance

In finance, mathematics is essential for risk assessment, portfolio optimization, and financial modeling. Financial analysts use mathematical tools to analyze market trends, predict future cash flows, and make informed investment decisions. For instance, the Black-Scholes model, a mathematical formula, is widely used to price options, a type of financial derivative.

General Inquiries

What are the fundamental building blocks of mathematics?

The fundamental building blocks of mathematics include numbers, variables, and operators.

How are building blocks used in mathematical operations?

Building blocks are combined and manipulated through mathematical operations, such as addition, subtraction, multiplication, and division, to form mathematical expressions and equations.

What are mathematical structures?

Mathematical structures are organized arrangements of building blocks, such as sets, groups, and rings, which possess specific properties and applications in various mathematical domains.